Future perfect

The sixth article in our series of schools briefs about finance looks at the apparently arcane world of derivatives and their importance to financial markets

IF YOU believe what you read in newspapers, derivatives (options, swaps and so on) are new, complex, risky and nasty—and of value only to those who make money selling them. In the mid-1990s, indeed, rapid growth in derivatives markets (not to mention some spectacular losses by those that used them) prompted concerns among regulators that derivatives might pose a threat to the global financial system.

In recent years these worries have abated, as regulators have become better acquainted with such instruments. Perhaps they should have learnt about them earlier. For although some (though far from all) of the mathematics used to calculate derivatives’ prices is fiendishly difficult, even the most complex can be broken down into two easily understood blocks: forward contracts, in which one party agrees to buy something from another at a specified future date for a specified price; and option contracts, in which one party agrees to provide the right—but not the obligation—to another to buy or sell something in the future.

Neither is exactly new. Aristotle mentions options in “Politics”; and there was an active market in tulip options in Amsterdam in the 17th century. In the same century an organised market for future delivery of rice was developed in Osaka. Even before that, traders at medieval fairs used arrangements that were recognisably forward contracts. Much of the arcane language merely reflects the fact that these two blocks are being applied and combined in different ways.

Forward markets are the simplest. In the markets for perishable commodities, their forward price is determined by expectations about future demand and supply. For financial assets, the price is determined by the cost of holding the asset. In foreign-exchange markets, for example, the forward rate is arrived at by looking at the difference between two currencies’ interest rates. Since no payments are made until the contract expires, the forward rate simply reflects the fact that one currency is paying higher interest. If it did not, somebody could buy the currency and sell it back at the same price, earning greater interest over the life of the contract. In other words, forward prices are not a market’s prediction about the direction a financial asset is likely to take.

Although a recent innovation (the first currency swap was between the World Bank and IBM in 1981), swaps markets are, in essence, like forward markets. The difference is that, in this market, the principal is paid back at the prevailing rate when the contract was signed (the spot rate); the two parties exchange interest payments, again so that one cannot make a riskless profit. In a typical interest-rate swap, for example, one party pays a floating rate for the duration of the contract, and the other a fixed rate. Swaps are, in effect, a string of forward rates—that is forwards that begin when the previous one finishes.

Options are different. They are a form of insurance. They enable a purchaser to buy or sell an asset at a certain price on a given date, but allow him to walk away if he wishes. The right to buy is generally dubbed a “call”, and to sell a “put”. There is also a difference between European-style options, which can be exercised (cashed in) only when they expire, and American-style ones, which can be exercised at any time during the option’s life. So, for example, if a person buys a European-style six-month call option on Citigroup, he would buy an option that has a six-month life, and can be exercised at the end of six months. If Citigroup’s share price falls in the meantime, he will not exercise the option.

Derivatives are traded in two types of market. The first is on exchanges, such as the London International Financial Futures and Options Exchange (LIFFE), or the Chicago Board of Trade (CBOT). The second is the over-the-counter (OTC) market—that is, people and firms trading directly with one another.

Exchange-traded derivatives differ in two main respects from OTC ones. The first is that exchange-traded contracts are almost always standardised: they try to make trading more liquid (and thus cheaper) by getting everybody to trade the same contract. For many, such liquidity outweighs some disadvantages, such as the fact that the instrument on which the contract is based might not be their preferred choice.

The second difference is that contracts are (usually) with the exchange’s clearing house, not with another bank, say. By guaranteeing that the contract will be honoured, the exchange removes so-called credit risk—highly pertinent for derivatives, because these are contracts to buy and sell things in the future and there is a risk, in the OTC market, of one of the contractors going bust or refusing to pay.

Clearing houses strip out this risk by asking users to stump up a deposit upfront (known as initial margin). They then ask for money from those that are losing on the contract and give it to those that are profiting. This is called variation margin. This mechanism, in essence, substitutes for credit risk, the risk that losers might have to fork out should their trades turn sour. In late 1993, an inability to find this margin nearly pushed Metallgesellschaft, a big German mining conglomerate, to the brink. The same fate befell Ashanti Goldfields, an African gold-mining firm, this year.

Most derivatives are variations of forwards and options, traded in different ways. A futures contract is really only a forward traded on an exchange. A swaption is an option on a swap. A futures option is an option based on a futures contract. A cap is the short-term interest-rate version of a call. Straddles are a strategy whereby somebody simultaneously sells a put and a call.

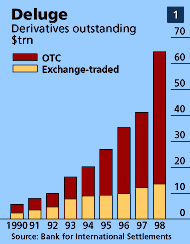Many things have contributed to the 12-fold increase since 1990 in the use of derivatives (see chart 1). Originally, the main demand came from farmers and those to whom they sold. Farmers knew their costs; to prosper (even to survive), they needed to know at what price they could sell their produce. The CBOT was set up as an agricultural exchange in 1848.

In recent decades, demand has come mainly from those who wish to hedge financial exposures. Financial markets became more volatile in the 1970s and 1980s. After the breakdown of the Bretton Woods system of fixed exchange rates in the early 1970s, exchange rates became much more volatile. This was exacerbated by the two oil shocks in that decade and by higher inflation—which also led to more volatile interest rates (see chart 2). Demand for instruments to guard against these risks surged.

The first currency futures were launched by the Chicago Mercantile Exchange’s International Monetary Market in 1972; and the first interest-rate futures by the CBOT in 1975. Since then, derivatives exchanges have sprung up in all developed economies and in many developing ones.

Demand comes from many quarters. Banks have used interest-rate derivatives to manage potential mismatches between their assets (loans and so forth) and their liabilities (checking accounts, for instance). Banks often have assets with a fixed rate of interest but pay a floating rate on their liabilities. To try to match these they could use interest-rate swaps, or they could purchase options that, for example, “cap” what they might be forced to pay out, or put a “floor” on the rate they would receive. Fund managers often use futures to protect against a decline in the value of their equity and bond portfolios. They might, for example, sell stock-index futures if they were worried that the stockmarket would fall: if it did, the gains from their futures would, with luck, offset the losses on their shares.

Companies also use derivatives to manage the risk that movements in the price of currencies and commodities might make their business uneconomic. Take the example of a British company exporting to America. If sterling rises too much against the dollar, its products might become too expensive. So it might try to protect itself by locking in an exchange rate using forward currency contracts, perhaps.

Derivatives are also commonly used by both financial and non-financial firms when they raise capital. A Japanese firm might want to borrow yen at a floating rate. However, there might be more demand for its debt from dollar-based investors who want to be paid a fixed rate. In that case it might be cheaper to issue fixed-rate dollar debt and use a currency swap (from dollars into yen) and an interest-rate swap (from fixed to floating interest rates) to achieve the desired result.

At the same time, financial firms have been able to satisfy (and often to create) demand because they have become better able to put a price on derivatives and to develop almost limitless variants, some of them highly complex.

Probably the most fundamental advance in financial economics this century occurred in 1973, when two financial economists, Fischer Black and Myron Scholes (with help from a third, Robert Merton), published a paper with the snappy title “The pricing of options and corporate liabilities”. This paper solved a riddle that many economists had failed to answer: what is an option worth? Take the example of a call option on a share. If the seller of the call buys the same amount of shares as he has granted the option buyer the right to buy, he runs the risk that the market will fall and the call buyer will not exercise his option. That would mean that the seller would be stuck with a loss-making holding. Calculating the worth of that option was little more than guesswork.

Messrs Black and Scholes provided an algorithm that worked out how to calculate an option’s worth. There are a number of inputs into their model: the current price of the underlying asset; the option’s strike price (the price at which the purchaser can buy or sell something); the level of interest rates; the time to maturity; and the volatility of the asset.

The last is the most important. The model does not try to predict in what direction the asset will move, but to calculate the risk of the option being exercised. This is best captured by seeing how volatile the asset is likely to be over the life of the option: the more volatile, the more likely it is that the option will be exercised—and the more expensive the option. Crucially, the model tells the seller how much of an asset a seller must buy or sell so as to cover his risks. This is called the hedge ratio or, more commonly, the option’s delta.

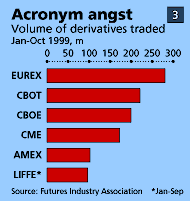
Two other things have helped the development of the options market—and, indeed, other derivatives. The first has been immense advances in computer power and a relentless decline in its price. The first pocket calculator, from Texas Instruments, also came on to the market in 1972. And, second, new exchanges have sprung up. The Chicago Board Options Exchange opened its doors in 1973; LIFFE began trading in 1982; Matif in Paris in 1986. The rivalry among these exchanges is intense (see chart 3). In the past two years, for instance, the floor-based LIFFE has lost European business to the screen-based Eurex, so that LIFFE has been forced to abandon its expensive floor altogether.

Do derivatives make the world riskier? Certainly, some companies that have used them have lost lots of money. Think, for example, of Procter & Gamble, which lost heavily in 1994 (as did several other firms) by using complex interest-rate swaps; or of Barings, a British bank that was felled in 1995 by the activities of an employee, Nick Leeson, in Japanese stockmarket futures and options markets.

Yet in such cases derivatives were not, of themselves, the main culprits. Either or both of two other problems were generally to blame. Many companies that lost money using derivatives treated their treasury departments, which deal with the cash coming into and out of a company and organise its borrowing, as a profit centre. This gave them an incentive to take risk. The second common fault has been bad risk management. In some cases—Barings is an example—this has opened the way for fraud.

Others argue that derivatives increasingly affect the instruments on which they are based—the tail wagging the dog. This argument, too, is wrong-headed. Derivatives are simply another, more efficient way of dealing in the underlying instrument (and swaps have no underlying instrument anyway); there is no tail and no dog, since it is all one market.

A more sophisticated argument is that derivatives encourage risk-taking because they have natural leverage; somebody can gain control of an exposure to, say, a bond in the futures market for a small fraction of the amount that it would cost if he were to buy the bond itself. But leverage is not a property unique to derivatives markets. Companies can and do gain just as much leverage by buying an asset and using it as collateral for a loan. California’s Orange County managed to bankrupt itself successfully by such means.

Might modelling be inherently risky? Or, to put it another way, do the models by which derivatives are priced have any bearing on what is actually happening in the real world? If they do not—and remember that derivatives are often long-term contracts—problems might build up unspotted. In fact, there is a market for bog-standard derivatives; to that extent it is easy to determine their correct price. The problem arises only for the small number of complex ones. Even then, it is unclear that it is worse than any other risk-management problem—putting your bad trades in the bottom drawer, for instance.

There is, however, one area in which derivatives might increase volatility. An option seller can hedge himself by buying another option or by buying or selling shares, say, in the market. In some cases, when everyone is trying to sell something at the same time, the market might be driven down and the model would then force investors to sell even more. This effect was widely blamed for the severity of the stockmarket crash in 1987. Under so-called portfolio insurance, by which investors had attempted to replicate the Black-Scholes hedge ratio for themselves (and thus cut costs), they were forced to sell increasing amounts of shares as the market fell.

Yet such effects aside, most of the academic studies that have looked at the question have found that derivatives do not make financial markets more volatile; many, indeed, have found that the opposite is true. Perhaps this should come as no surprise. Derivatives are really only an efficient way of transferring risk from those that do not want it to those that do. If risk is in safer hands, the net effect ought to be to reduce volatility. That’s not so nasty, is it?

Copyright © 2008 The Economist Newspaper and The Economist Group. All rights reserved.